Population Size
Populations are fluid and are subject to continual change in numbers through natality, mortality and migration
-
Populations may be large and impractical to count unless the species is large and the target area is small
Population sampling involves identifying individual numbers in small areas and then extrapolating to estimate population totals
-
The difference between the estimate of population size and the true size of the whole population is known as the sampling error
-
The more samples that are taken (and the larger the sampling area), the more accurate population estimates are likely to be
In random sampling, the positions of the sampling points are selected at random to avoid the potential for bias
-
Sampling sites can be randomly selected by establishing a grid of the target area and arbitrarily assigning grid coordinates
In systematic sampling, the positions of the sampling points are assigned at fixed intervals throughout the target area
-
This allows for the investigation of the effect of a changing environmental factor on species distribution (via a transect)
Population Sampling

Random Sampling
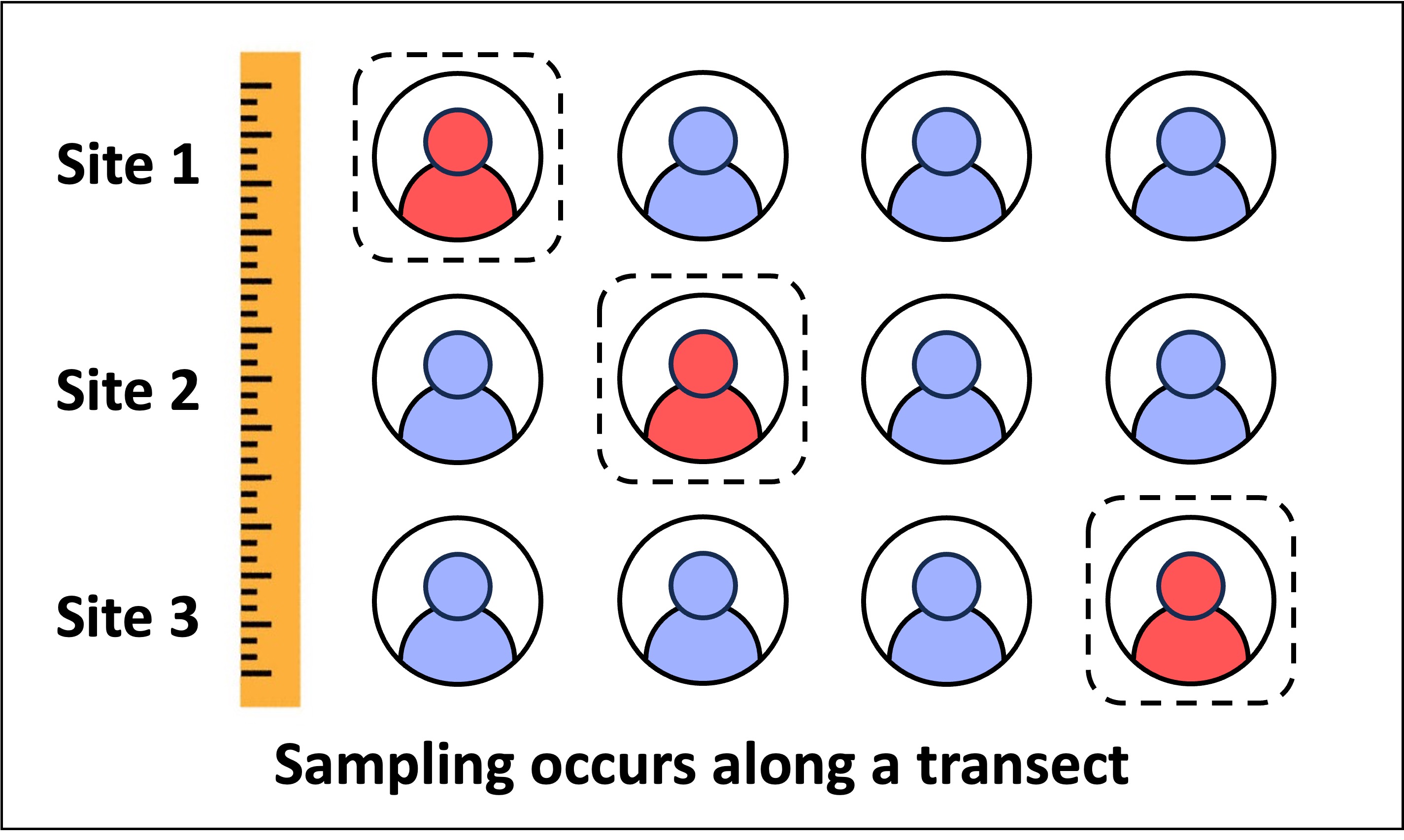
Systematic Sampling
Quadrat Sampling
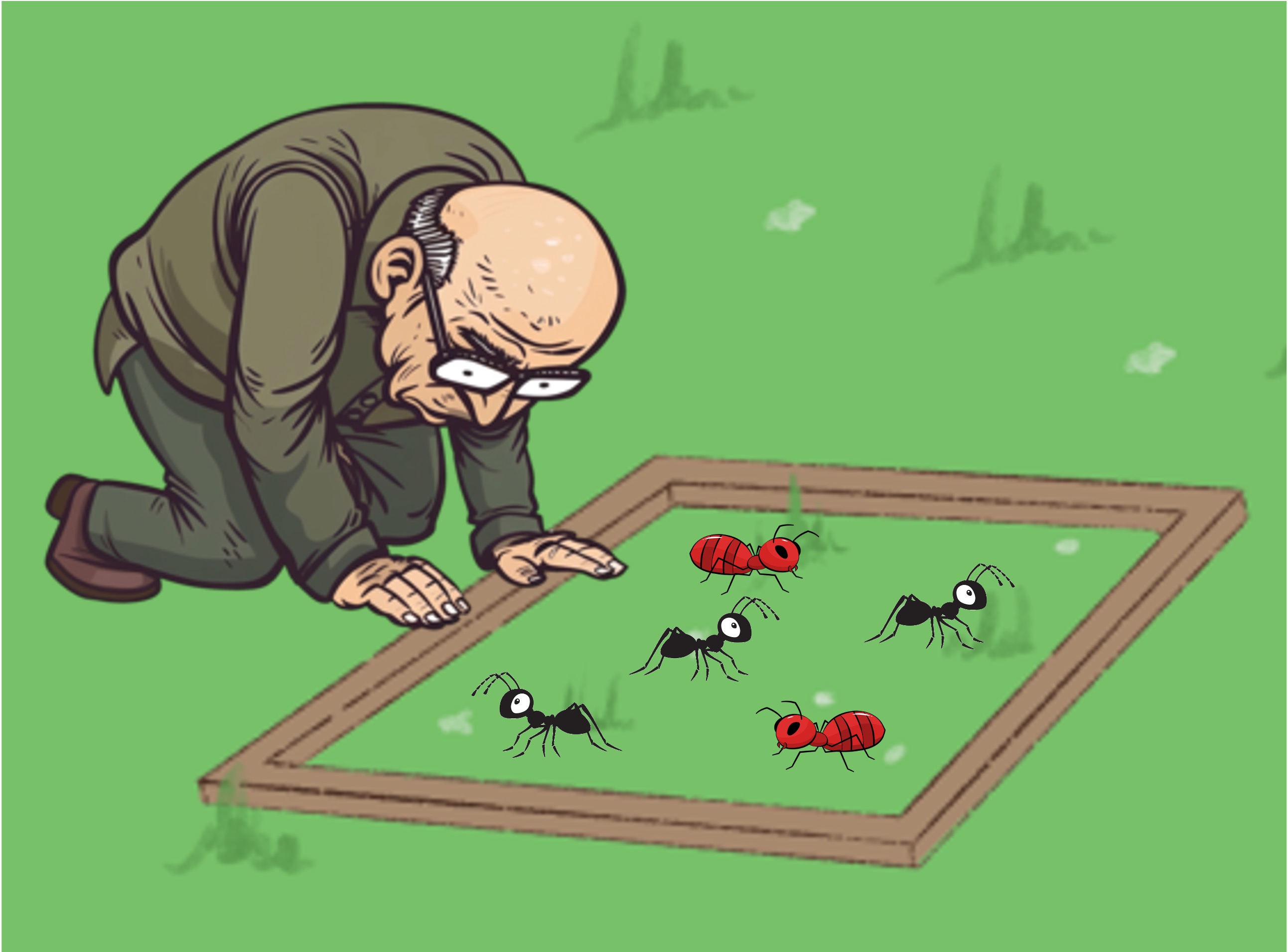
The population size or distribution of a non-motile (sessile) species can be determined using quadrat sampling
-
A quadrat is a rectangular frame of known dimensions that can be used to establish population densities
-
Quadrats are placed inside a defined area in either a random arrangement or according to a transect
-
The number of individuals of a given species is either counted or estimated via percentage coverage
If the sampling process is repeated many times, a mean and standard deviation can be calculated
-
The standard deviation shows the degree of data spread and could be used to indicate how evenly distributed the population is across the habitat
Counting Sessile Species
Lincoln Index
The population size of a motile species can be determined using the capture-mark-release-recapture method
-
This method involves the collection of three pieces of data which are used to derive a population estimate:
-
M: The number of individuals that are captured within a defined area, then marked and released
-
N: The number of individuals that are recaptured after sufficient time has passed for reintegration
-
R: The number of marked individuals in the recaptured sample
-
The Lincoln index is a formula that provides a population size estimate based on three collected values
-
Population Estimate = M × (N ÷ R)
The Lincoln index requires that the following assumptions are true:
-
That all individuals in a given area have an equal chance of being captured (sampling must be random)
-
That marked individuals will be randomly distributed after release (reintegration allows for an even spread)
-
That the action of marking individuals will not affect the mortality or natality of the population
-
That the marking will remain visible for the duration of the sampling process (not removed before recapture)
-
That the population size does not change significantly between the periods of first and second capture
Counting Motile Species
Capture and Mark